Please 'Boom' Responsibly As most of you have noticed, the noise ordinances have become much tougher lately. Most of this is due to idiots, yes IDIOTS, who drive through residential areas with their windows down while their system is playing at full power. To make things worse, the music they listen to has all sorts of foul language that's not suitable for small children, (who may be playing outside). There are even a few people, who are even beyond idiot status, that play their systems at full power through residential areas after 10:00 PM (when many people go to bed). I don't believe that this type of behavior is good for the industry. If the fines get too stiff, people will stop buying large systems. If this happens, more people will get out of car audio (who wants a mediocre system). People get interested in things because they're exciting. A deck and four 6.5" speakers are not going to interest many of the younger car audio enthusiasts. If car audio enthusiasts keep annoying more and more people, the fines will keep getting tougher. All of this will only reduce interest in the equipment that fuels the industry. If you want to listen to your system at full volume, get out on the highway where there's little chance of bothering anyone. When you get to a red light, turn it down. If the only thing attractive about you is your 'system', you have some work to do. Bottom line... Think about what you're doing. Think about other people. It's not the end of the world if you have to turn the volume down for a little while. |
|
| |
Click HERE to make the calculator fill this window.
| Loss of Power Output: Since amplifiers are relatively inefficient and can draw significant amounts of current, it's necessary to use the right size wire. The following demo shows how much voltage and power is lost with a given wire size. Notice that there is loss in the ground wires also. All of the voltage lost across all of the conductors are added together to give the total loss. That's subtracted from the battery (charging system) voltage. What's left is what the amplifier sees. Notice that the loss is not constant. It's proportional to the power output of the amplifier. At zero volume, there is virtually no current draw and no loss of voltage (battery_voltage=amplifier_voltage). When the amplifier is at or near full power, the drop in voltage is much more significant. Length is in feet. 'Gauge' is American Wire Gauge. |
| |
Click HERE to make the calculator fill this window.
Quick Reference: This table shows the amount of current flow which will cause a 1/2 volt drop in a 15 foot run of cable. Many people consider 1/2 volt to be the maximum acceptable voltage loss in a system's main power wire. The 'total amp power' is the total maximum unclipped RMS power output‡ of all of the amplifiers combined. It is based on 60% efficiency (for class AB amplifiers) and a battery voltage of 13.8 volts. ‡ For all of the calculators and tables on this page, unless otherwise noted, 'max power' is the RMS power output when the amplifier is on the threshold of clipping. |
|
| Wire Gauge | Recommended Maximum Fuse Size |
| 00 awg | 400 amps |
| 0 awg | 325 amps |
| 1 awg | 250 amps |
| 2 awg | 200 amps |
| 4 awg | 125 amps |
| 6 awg | 80 amps |
| 8 awg | 50 amps |
| 10 awg | 30 amps |
| 12 awg | 20 amps |
| 14 awg | 15 amps |
| 16 awg | 7.5 amps |
| These are the recommended maximum fuse ratings for the corresponding wire size. Using a smaller fuse than what's recommended here will be perfectly safe. |
| Please note that the
recommended fuse ratings are roughly based on 300
circular mils (explained at the bottom of the page) of
copper per amp of current. Others may suggest slightly
different fuses for a given wire size but these should be
generally recognized as safe in most all situations. Maximum Power for a Given Wire Size: If you have a power wire in your vehicle and want to know how much power you can run on it, use the following calculator. If you don't know if you have class A/B or class D amplifiers, leave the efficiency at 50%. |
| |
Click HERE to make the calculator fill this window.
| Wire and Fuse Selection: The following calculator will give basic wire and fuse sizes for the main power wire and for each individual amplifier. Select the class of operation for each amplifier and enter the power output at the right of the amplifier. If you have less that 4 amplifiers, enter zero in the fields that are not needed. Keep in mid that these are just suggestions. If your amplifiers don't have internal or on-board fuses, use the manufacturers suggested fuse in the distribution block. The fuse sizes given are to protect the vehicle from fire not necessarily to protect the amplifier. |
| |
Click HERE to make the calculator fill this window.
Power Wire Calculator
|
|
|
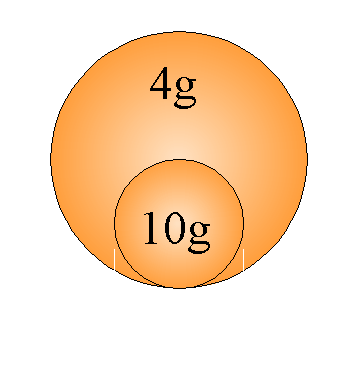 |
This image shows the relative difference between 10g and 4g wires (not actual size). You can easily see that doubling the diameter quadruples the cross sectional area. |
|
| or 1 square mil = 1.273 circular mils |
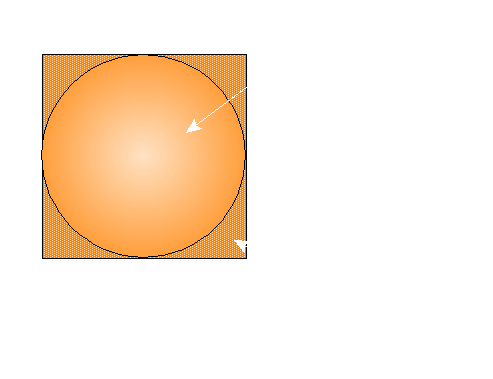 |
This diagram should help you understand the difference between a circular and a square mil. |
|
|
|
|
|
||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
Wire Insulation Types
|
| T | Thermoplastic insulator (generally PVC) |
| H |
|
| N | Nylon outer insulator (protects against abrasion) |
| S |
|
| B | Braided |
| W | Wet locations |
| R | Rubber (non specific) |
| RU | Latex rubber |
| A | Asbestos |
| F | Fixture Wiring |
Specific Wire Designations (individual conductors)
| THHN | High temperature (90°C 194°F max.) thermoplastic for use in dry locations like building wiring with a nylon outer insulator. |
| THWN | Standard temperature (75°C 167°F) thermoplastic for use in wet or dry locations with a nylon outer coating. |
| RW | Moisture resistant rubber |
| R | Rubber |
| RH | Rubber moderate temperature (75°C 167°F) |
| RHH | Rubber high temperature (90°C 194°F) |
| TBS | Thermoplastic insulator with braided cover (generally used for switchboard applications) |
| RUH | Heat resistant latex rubber |
You should remember:
1.Wire has resistance and therefore will have a voltage drop
across the length of it any time current is flowing through it.
| If you find a problem
with this page or feel that some part of it needs
clarification, E-mail me.
This is a link to this site's home page. |