- 3 voltages- 1 sine wave
- In one of the earlier examples,
the power output of an amplifier was expressed as
peak power. The voltage used to determine the
power output was peak voltage. The next section
will explain the different ways to measure
voltage.
- Choices:
- There are 3 ways to quantify
the magnitude of a sine wave.
- Peak Voltage:
- Peak voltage tell you how far
the voltage swings, either positive or negative,
from the point of reference. Peak voltage is only
a moderately useful way of measuring voltage when
trying to express the amount of work that will be
done when driving a specified load. Some
manufacturers use peak voltage to get the power
output ratings of their amplifiers. I will
explain this in more detail a little later.
- Peak-Peak Voltage:
- It is rarely used. It is
probably more useful in the case of a
non-symmetrical wave form. Otherwise you would
probably express it's value as peak voltage.
- RMS Voltage:
- RMS voltage is absolutely the
most common way to measure/quantify AC voltage.
It is also the most useful. Because AC voltage is
constantly changing and is at or near the highest
and lowest points in the cycle for only a tiny
fraction of the cycle, the peak voltage is not a
good way to determine how much work can be done
by an AC power source (e.g. your amplifier, a
wall outlet in your house...). DC voltage is
constant. Its voltage level can be plugged
directly into the formulas for power (on the Ohm's law page) and you will get an accurate
image of its ability to do work. RMS voltage will
give you the same ability to predict how much
work will be done by an AC voltage. The RMS
voltage of a pure‡
sine wave is approximately .707*peak voltage. If
you read voltage with a voltmeter you are
generally given the RMS voltage of the wave form.
Some meters display an 'average' voltage which is
very close to RMS. When reading voltage with a
voltmeter, the display indicates the RMS or
average voltage not the peak or peak-peak
voltage.
- ‡If the
waveform isn't a pure sine wave (like a square
wave or a signal with mixed sine waves of
different frequencies or music), multiplying the
peak times .707 will not give an accurate RMS
value and therefore will not give an accurate
indication of the work that the waveform can
produce when driving a load. For more complex
signals, you need a meter that will calculate the
RMS value from a set of samples taken at regular
intervals. One such device (the TRUE RMS
voltmeter) is discussed below.
- More on RMS Voltage:
- In the following diagram, the
'filled' area is where the sine wave will do some
work (motivating speakers, etc.). The amount of
work that it will do at any given point in time
is determined by the magnitude (above and below
the reference) of the voltage (with reference to
the red line).
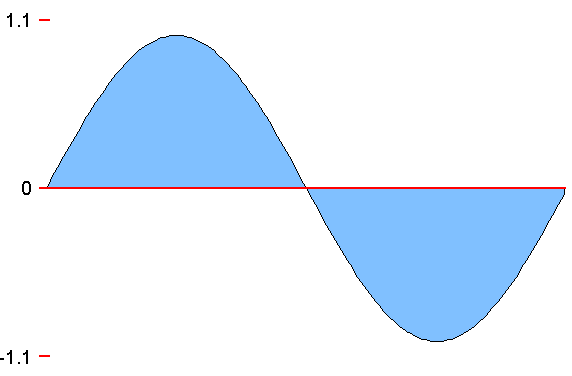
If you
look at the the blue area of the
diagrams, this is equal to this  . The 'negative'
voltages were simply converted to
positive voltages (for clarity). The
green area is the equivalent DC voltage.
As you can see in the diagram below, for
the same period of time, the AC voltage
is sometimes lower and sometimes higher
than the DC voltage. The amount of work
that can be done by the voltages in this
diagram is the same. Of course when I say
they are equal in the amount of work they
can do, I am talking about something like
heating a heating element or driving an
electric motor and not audio reproduction
(audio is an AC waveform). Note that the
DC voltage is roughly equivalent to 71%
of the peak AC voltage. |
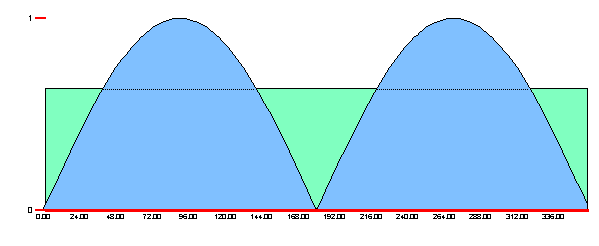
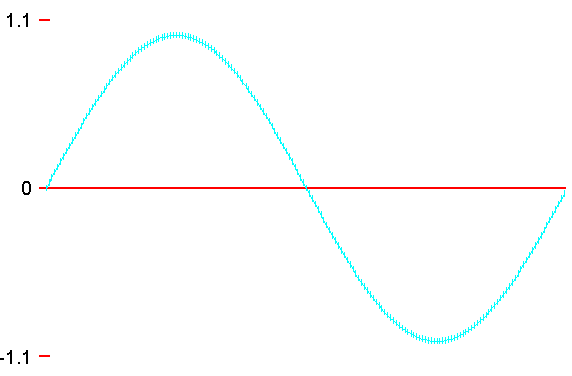
- Calculating Actual RMS Voltage:
- If you have a 'true RMS'
voltmeter, the meter measures the instantaneous
voltage at regular time intervals. On the
following graph, the little vertical lines along
the sine wave represent the points in time where
the voltage is measured. The microprocessor in
the voltmeter then 'squares' all of the voltages
at each point and adds the squared values
together. It then calculates the average (mean)
from the squared values. And finally... it
calculates the square root of the average (mean)
value.
- The following is a sample of
the data that's used to calculate the true RMS
voltage of a 1 peak volt
sine wave. The data is taken at 20º intervals (about 1/10 the data
points shown on the previous diagram). As you can
see the values come out to the same value as when
you multiply the voltage (1 volt in this case) by
0.707 (0.707 = 1/square root of 2).
- Warning:
- A word of caution, many
manufacturers of car audio can exaggerate their
power ratings by using peak power (derived by
using peak voltages). Peak power is twice as much
as RMS power and is not indicative of the actual
amount of work that the amp will do (driving your
speakers).
RMS
& Continuous Power output
- CONFUSION:
- Many people wrongly believe
that the RMS power rating is the same as a
continuous power rating.
- Power:
- First, power is a 'snapshot' of
the amount of work being done at any point in
time. It has no specified time component.
- Ratings:
- There are a lot of different
ways to rate an amplifier's output power
capabilities.
- A few possible ways to rate
amplifiers: Watts (not much information and lends
itself to many different interpretations)
- When manufacturers state the
power output in 'watts' (and only 'watts' i.e. 50
watts), they want you to assume that the wattage
rating is an accurate measurement of the
amplifiers ability to do a given amount of work
(driving your speakers). In reality, the power
rating could be derived by using peak voltage
instead of the more honest RMS voltage when
plugging the numbers into the formula P=E^2/R.
This would give a power output/rating that's
TWICE the RMS power output. Actually they could
be using virtually anything to derive this vague
specification (pulling a number out of thin air
comes to mind).
Peak watts (misleading
because many will take it as a true measure of
the work that the amplifier will do when driving
the speakers)
- If the manufacturer specifies
wattage as 'peak power' they may say that they
aren't trying to mislead their customers, but I
believe that that is precisely what they are
trying to do. They assume that the vast majority
of people are going to accept the wattage as the
maximum power that the amplifier can produce. If
they use peak voltage to derive the peak power,
the power specification may be legitimate but it
is misleading. The peak power is mathematically
twice the RMS power output.
RMS watts (Better
than peak power but it also lends itself to
misinterpretation because it contains no
specified element of time)
- RMS wattage is an accurate way
to measure power but the amplifier may only be
able to produce the RMS voltage into the given
load for a fraction of a second. The, less than
honest, manufacturer may plug this RMS voltage
into the P=E^2/R formula and give this number as
the amplifier's output. If they give this as the
power output of the amplifier but don't tell you
that the amplifier can't continuously produce
this power level, they are again misleading you.
RMS continuous watts (the
best, most accurate, honest way to measure power
output)
- When manufacturers rate their
amplifiers at a given number of RMS watts
continuous power output. They are using RMS
wattage (derived from RMS voltage) which is the
most accurate way to state the useful power
produced by the amplifier. There is only one way
to interpret this. They are also stating that the
amplifier can produce the power continuously. If
they didn't specify continuous power they may be
trying to cover up the fact that the amplifier
could only produce the RMS voltage required to
drive the given load to a given RMS power for a
fraction of a second (like in the previous
example). When they state continuous output
power, they are saying that the amplifier can
easily and continuously produce the rated output
power.
For the
Purists...
- RMS Power (PRMS):
- Throughout this site I will use
the term 'RMS power'. Technically, as far as I
know, there is no such thing as 'RMS' power. This
section will help clarify and define a few terms.
I will define RMS power as the power that's
calculated when using an RMS voltage or current
into a resistive load. Other ways to quantify
power...
- Average power (Pave):
- Average power is defined as the
RMS
voltage multiplied by the RMS current.
- Peak power (Ppeak):
- Peak power is defined as the peak voltage multiplied by the peak current.
|
