Please 'Boom' Responsibly As most of you have noticed, the noise ordinances have become much tougher lately. Most of this is due to idiots, yes IDIOTS, who drive through residential areas with their windows down while their system is playing at full power. To make things worse, the music they listen to has all sorts of foul language that's not suitable for small children, (who may be playing outside). There are even a few people, who are even beyond idiot status, that play their systems at full power through residential areas after 10:00 PM (when many people go to bed). I don't believe that this type of behavior is good for the industry. If the fines get too stiff, people will stop buying large systems. If this happens, more people will get out of car audio (who wants a mediocre system). People get interested in things because they're exciting. A deck and four 6.5" speakers are not going to interest many of the younger car audio enthusiasts. If car audio enthusiasts keep annoying more and more people, the fines will keep getting tougher. All of this will only reduce interest in the equipment that fuels the industry. If you want to listen to your system at full volume, get out on the highway where there's little chance of bothering anyone. When you get to a red light, turn it down. If the only thing attractive about you is your 'system', you have some work to do. Bottom line... Think about what you're doing. Think about other people. It's not the end of the world if you have to turn the volume down for a little while.

Series vs Parallel:
There are 2 ways to connect multiple devices to a power source (e.g. speakers to an amplifier), series and parallel. Well... OK, there's also series/parallel. But we'll cover that on a later section.

Resistors in series

Speakers in series
Speakers connected in SERIES

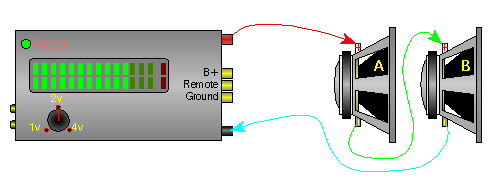
In a series circuit (like the two above), the current must flow through one device to get to the next device. This means that the rate of current flow through all devices is the same. The voltage across each device depends on its impedance/resistance of each device and the current flowing through the circuit. When adding more components in a series circuit, the current flow decreases, if the applied voltage remains constant.

Resistors in parallel

Speakers in parallel
Speakers connected in PARALLEL

In a parallel circuit (like the two examples above), each device is directly connected to the power source. This means that each device receives the same voltage. The amount of current flowing through each device is dependent on the impedance/resistance of that particular device. If devices are added to the power source in a parallel configuration, the current demand/flow from the power source increases.
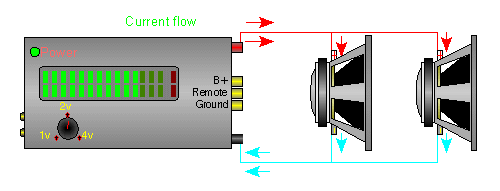
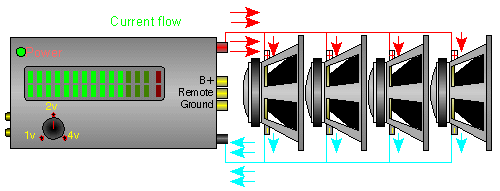
In the 2 diagrams below, you can see the relationship between the current flow out of the amplifier and the number of speakers. You can see that four speakers draws twice as much current from the amplifier than the two speaker configuration.

Current flow is twice the current flow of 1 speaker.

Current flow is 4 times the current flow of 1 speaker.

When making any connections to any power source you must know the limits of the source, to prevent damage to the source. This means that if you connect too many speakers, in a parallel wiring configuration, to an amplifier (the power source in this case) it may well be damaged beyond repair. I've seen it happen, especially when the amplifier was improperly fused.

For those Who Want to do the Math...

In the diagrams/text above, we had either series OR parallel circuits. This section will show you what happens to the voltage, current and power dissipation in a series/parallel circuit. As was said before, in a series circuit, the 'current' in each device is the same. In a parallel circuit, the 'voltage' is the same across each device. In the following circuit, you can see that there are two 1000 ohm resistors in series with a single 400 ohm resistor. We know that the voltage across the two 1000 ohm resistors is going to be the same (because they're connected in parallel). We also know that the total current flow through the two parallel connected resistors will equal the current flow through the 400 ohm resistor.

To calculate the total current, we should first simplify the circuit. This means we need to find the total resistance of the parallel network. For a simple circuit with two equal value resistors, we can simply divide the resistance of a single component by the total number of components. For this parallel network, we have two 1000 ohm resistors. If we had 3 parallel resistors, we'd divide 1000 by 3 to find the total resistance of the parallel resistors.
Total resistance of parallel resistors = resistance of a single component/number of resistors
Total resistance of parallel resistors = 1000/2
Total resistance of parallel resistors = 500 ohms
 
Now that we know that the parallel resistors are equal to a single 500 ohm resistor. Now that we have, esentially, one 500 ohm resistor in series with a 400 ohm resistor, we can calculate the total current through the circuit. We know that we have a 12 volt power source. We also know that the 500 ohm resistor in series with the 400 ohm resistor is equal to a 900 ohm resistor.
Current flow through circuit = voltage across circuit/total circuit resistance
Current flow through circuit = 12/900
Current flow through circuit = 0.0133 amps
 
Now we can find the voltage across the individual components. To reduce the possibility of getting confused, calculate the voltage across the single resistor first.
Voltage across the resistor = resistor's resistance*current flow through resistor
Voltage across the resistor = 400*0.0133
Voltage across the resistor = 5.333 volts
 
In a series circuit, all of the voltages across all of the individual series components will equal the power supply voltage. If we have a 12 volt source and the voltage across the 400 ohm resistor is 5.333 volts, we know that the voltage across the parallel pair of resistors is going to be 6.67 volts (12-5.333=6.67). To calculate the current through the parallel components...
Current through a single 1000 ohm resistor = voltage across resistor/resistance
Current through a single 1000 ohm resistor = 6.67/1000
Current through a single 1000 ohm resistor = .0067 amps
 
Now that we know the voltage across each of the components and the current flow through each of the components, we can calculate the power dissipation for each component. Actually we could have done it as soon as we knew the voltage across the components but I decided to take the 'scenic' route.
Power dissipation in the 400 ohm resistor = voltage across component*current through component
Power dissipation in the 400 ohm resistor = 5.33*0.013
Power dissipation in the 400 ohm resistor = 0.071 watts
 
Power dissipation in each 1000 ohm resistor = voltage across component*current through component
Power dissipation in each 1000 ohm resistor = 6.67*.0067
Power dissipation in each 1000 ohm resistor = .045 watts
 
This example used resistors but the same calculations would work for any resistive device. Although speakers are not purely resistive when being driven with AC voltage, the calculations here could be used to make rough calculations for voltage, current and power dissipation in speakers.

And a calculator to check your math if you want to try the above circuit with different values.
Input
Power Supply Voltage X? Volts
Power Supply Voltage Y? Volts
Resistor A Value? Ohms
Resistor B Value? Ohms
Resistor C Value? Ohms
Output
Total Power Supply Voltage Volts
Total Power Supply Current Amps
Total Resistance a||b Ohms
Total Resistance a||b+c Ohms
Voltage Resistor A Volts
Current Resistor A Amps
Power Dissipation Resistor A Watts
Voltage Resistor C Volts
Current Resistor C Amps
Power Dissipation Resistor C Watts
Voltage Resistor B Volts
Current Resistor B Amps
Power Dissipation Resistor B Watts

For more information on series/parallel circuits and the impedance of different speaker loads, go here.
 

You should remember:
1.In a series circuit, the same current flows through all of the series connected components.
2.In a parallel circuit, the same voltage is applied to all of the parallel connected components.

backward If you find a problem with this page or feel that some part of it needs clarification, E-mail me.

This is a link to this site's home page.

forward